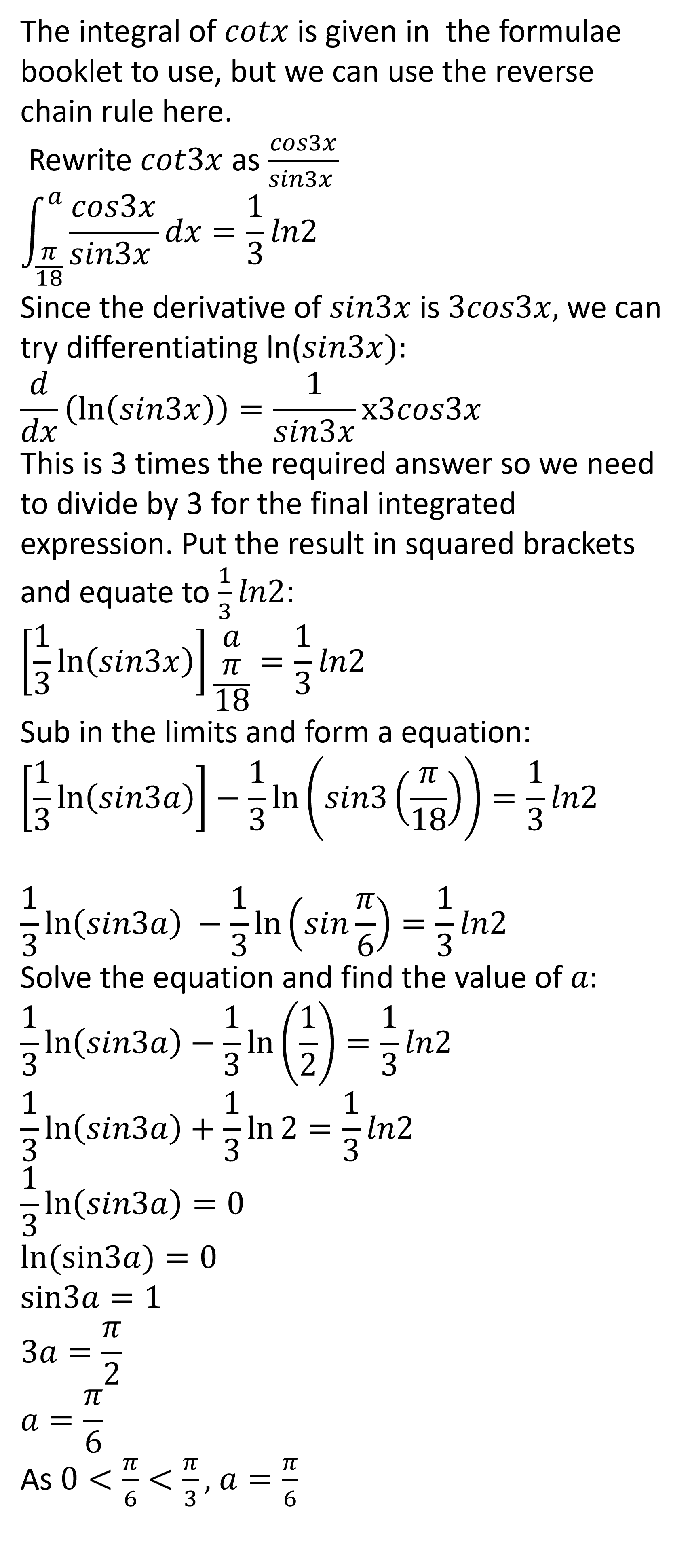
Reverse Chain Rule
We can integrate expressions in the form f(ax+b) by doing the reverse of the chain rule. Generally you can use the following formula to find these types of integrals:
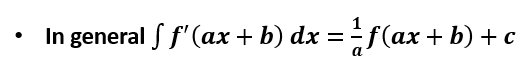
Example 1:

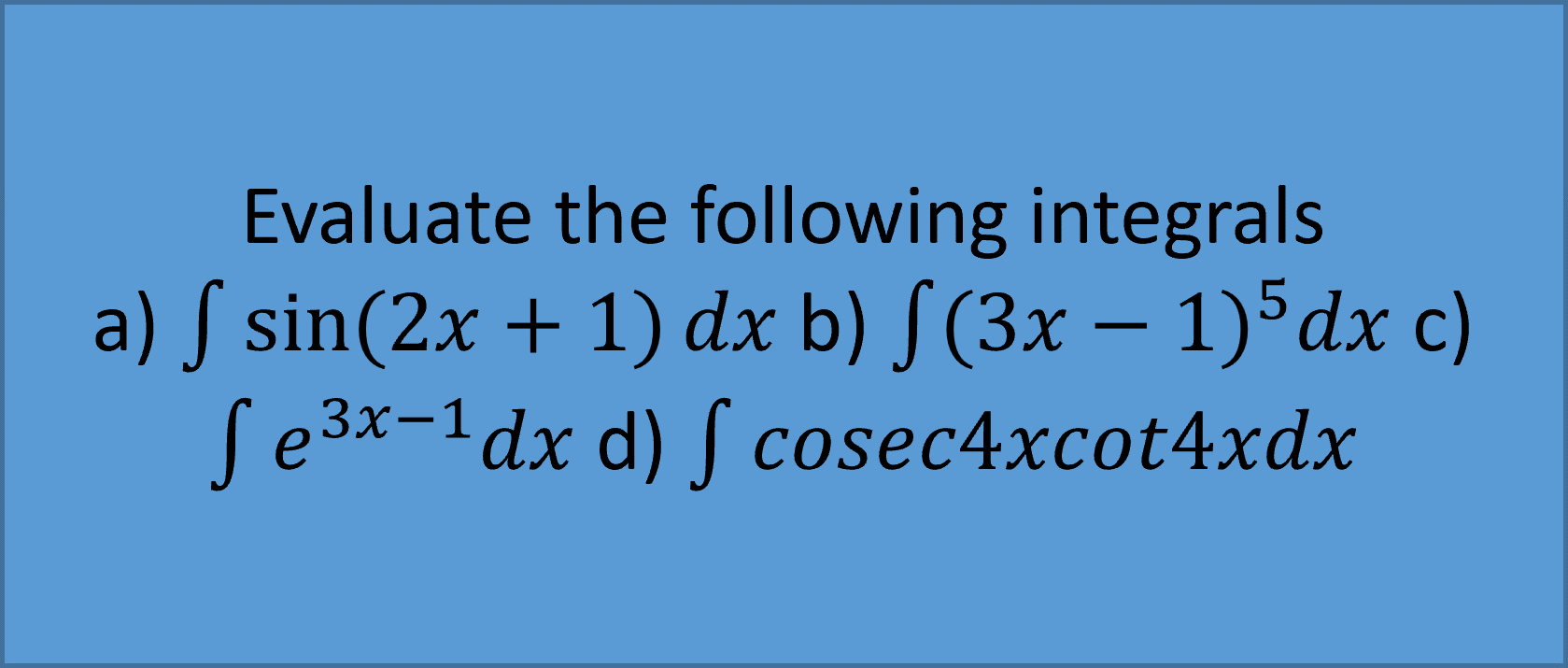

Note: You can simply use the formula straight away for cases similar to the parts in example 1, but it is important to understand the process behind how to get the final answer in order to tackle more difficult cases and cases that require more steps which will be seen below. Then you will be able to solve this integrals intuitively.
You will come across cases in the following form:

This concept behind this case is that if you differentiate an expression with ln|f(x)| using the chain rule to get f'(x)*1/f(x) and readjust the constant, you should be able to get the original expression.
Example 2:


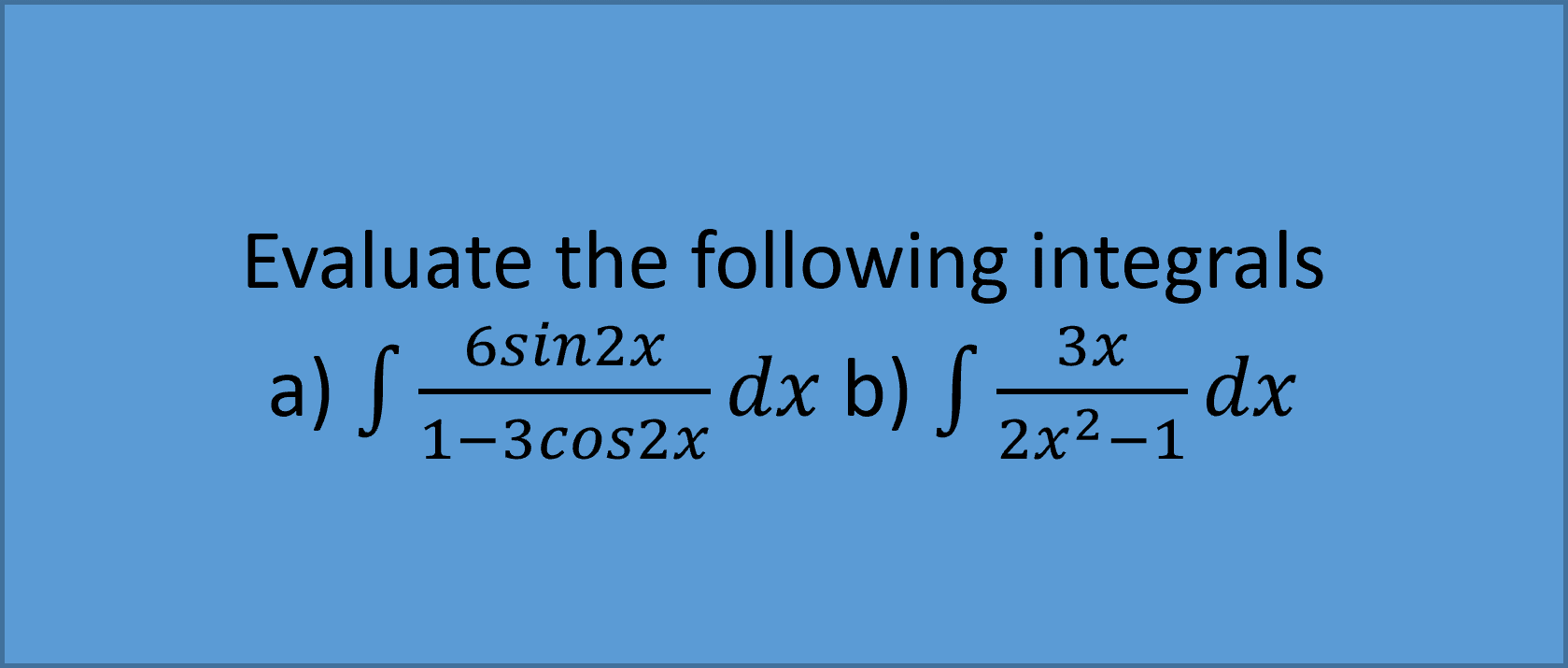

One last case where you will need to try to use the reverse of the chain rule is:
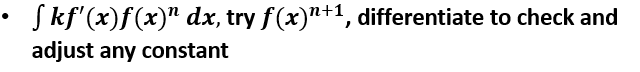
This concept behind this case is that if you differentiate an expression with f(x)^(n+1) using the chain rule to get f'(x)*(n+1)f(x)^n and readjust the constant, you should be able to get the original expression.
Example 3:
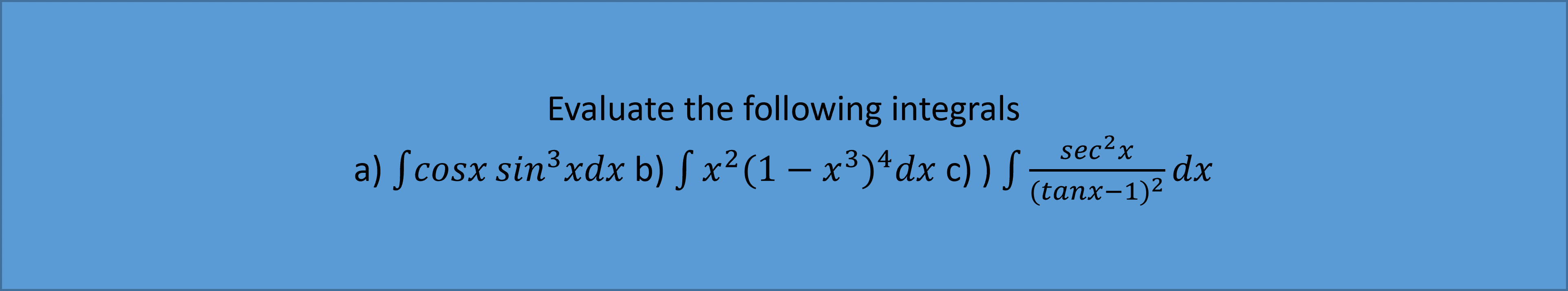
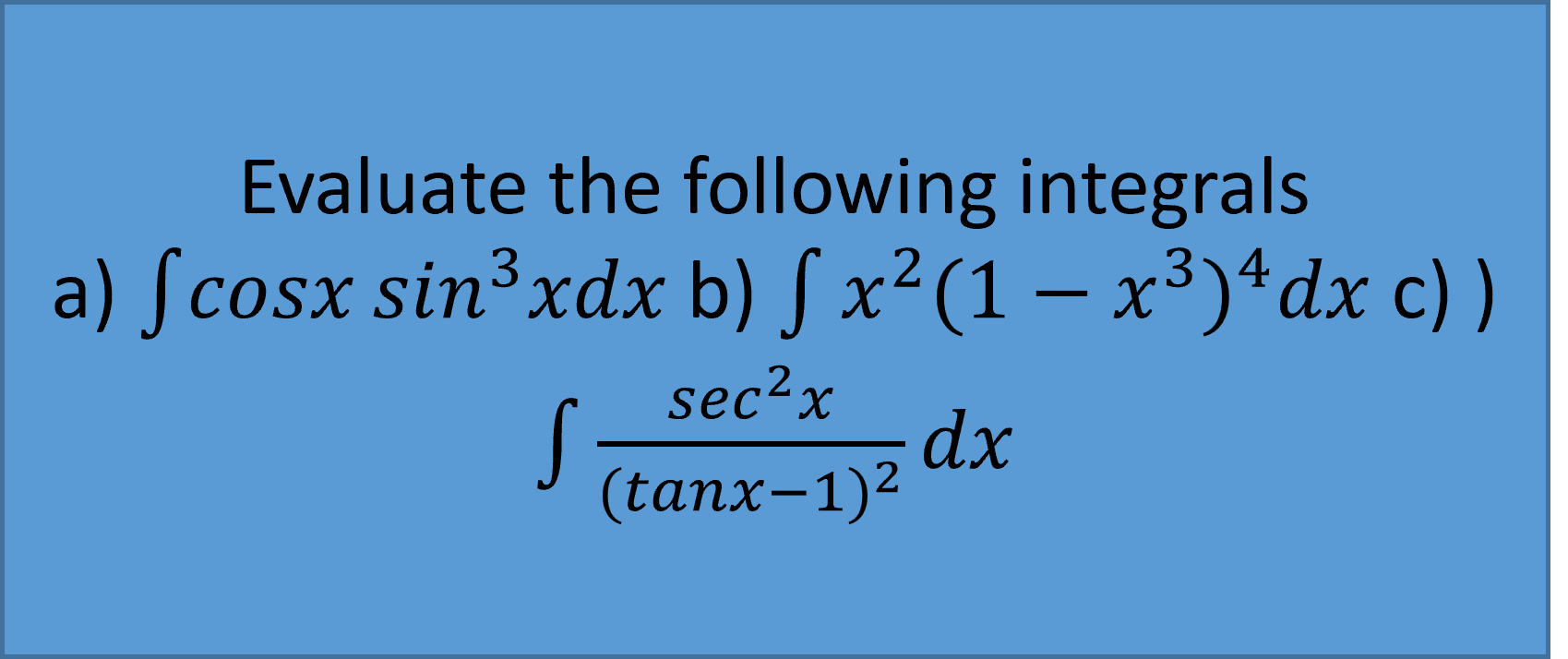

Note: The very last part in example 3 looks very similar to the previous type of case with ln|f(x)| but it can only be solved in the way shown in the example. To differ between both cases, check the expression f(x) and ensure that if f(x) is not to the power of n where n>1 and it is in the denominator of the expression, you use the case with ln|f(x)|. Otherwise you use the next case.
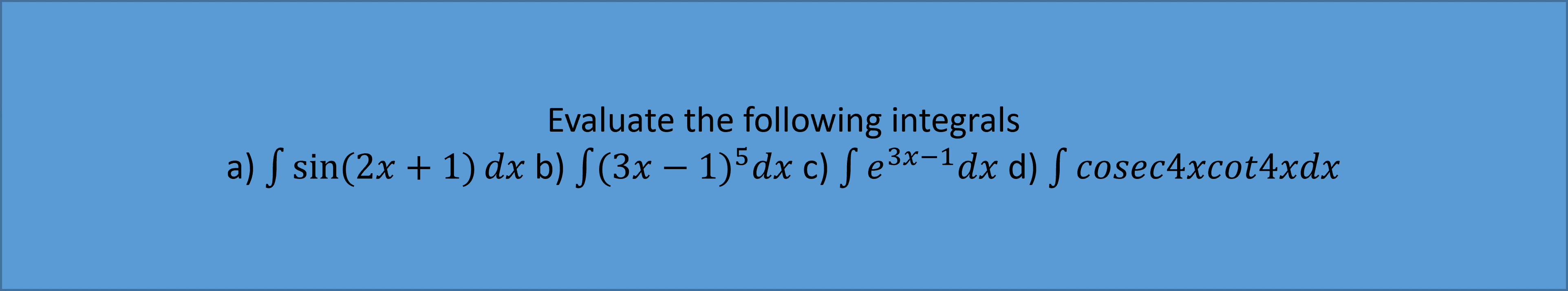
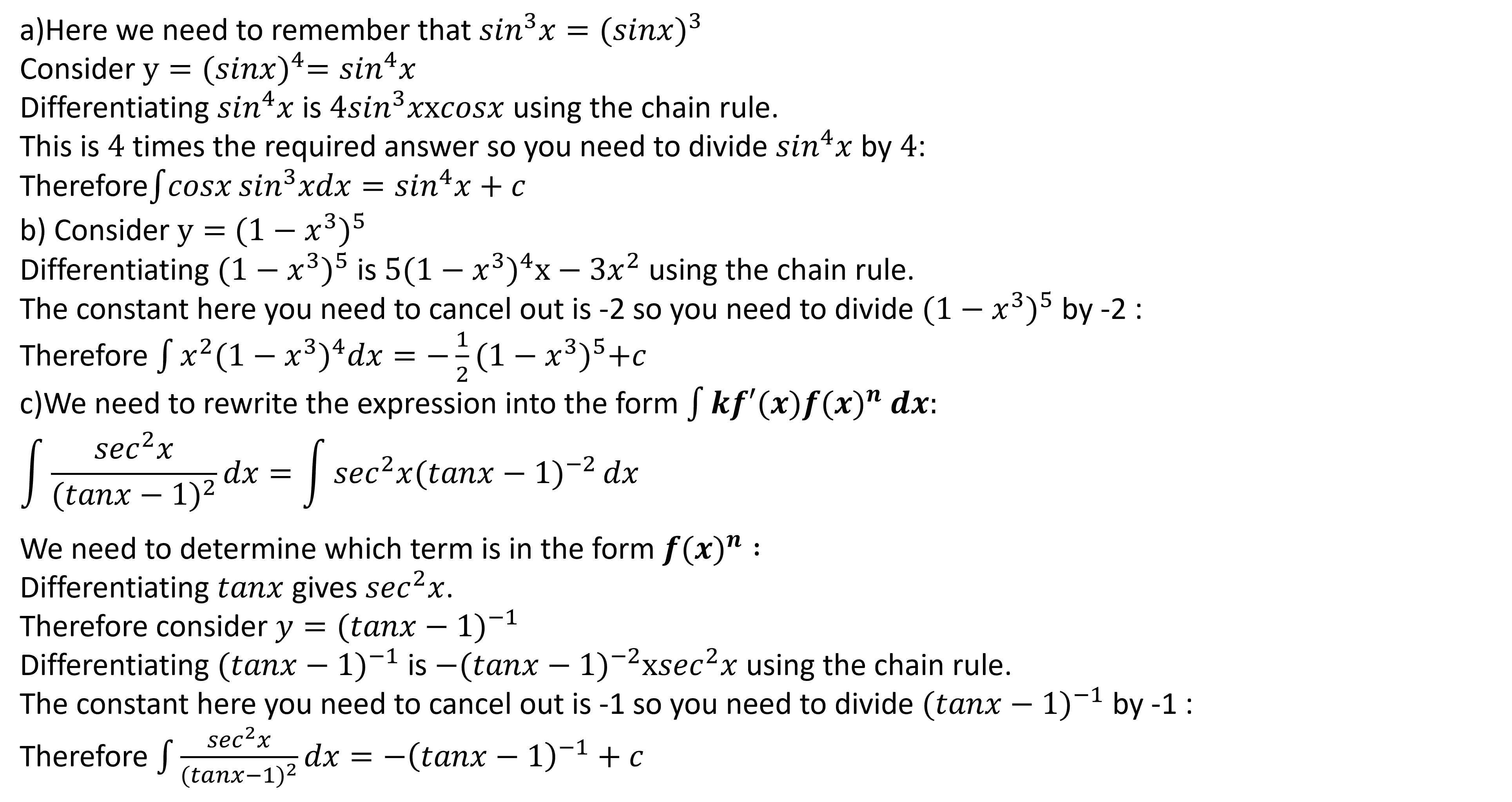
Practice Question: